LSAT Forum
2 postsPage 1 of 1
-

- aebq196234
-
Thanks Received: 2
- Forum Guests
- Posts: 13
- Joined: August 01st, 2012
Q20
i am having difficulty understanding the question stem. what exactly does this mean?
-

- timmydoeslsat
-
Thanks Received: 887
-
Atticus Finch

- Posts: 1136
- Joined: June 20th, 2011
- This post thanked 1 time.

- Most Thanked

- First Responder
Re: Q20
We are told of a condition where the people who vote against school and against tax are the exact same people. We are asked what also must be true with this condition.
This question could be solved by looking at a global diagram. Mine is below:

For the S and T columns to be exactly the same, I must place an X for S-H and for T-F.
This would force me to place a checkmark for T-G, as I know that exactly one person votes for G, and there is only one person left. This would then force S-G to be a checkmark to keep with the condition of the question.
That is one way to solve it. Or you could, as I did from the beginning, frame the game. You know it has such limited options.
From the global diagram, as shown above, you can see that the R column must contain exactly 2 checkmarks, and we only have one person left to vote, which means R-H must have a checkmark.
Knowing that we have a constraint that each voter must have at least one checkmark and at least one X, we can see that only voter G has potential issues with this requirement, as everybody else has both checkmarks and X's. At this point, I would frame the game around S-G being a check mark and S-G being an X.
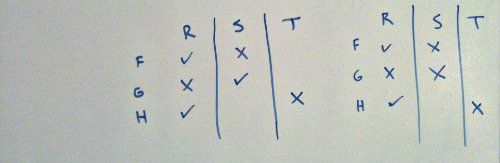
If you use the global constraints concerning the alottment of checkmarks and X's each column has, you can quickly infer that in the left hypothetical, S-H must be an X. In this same hypothetical, we only have two spaces left to figure out. We know one must be a checkmark and one must be an X. However, all of the other requirements have been met, which involved each voter having at least a checkmark and an X, so this means that we can have a dual option amongst these two variables.
In the right hypothetical, we can quickly infer two things. We know that the G row must have at least one checkmark, so this means that T-G will have a checkmark. We also know that the S column has exactly one checkmark, and we only have one voter left, so S-H is a checkmark. We have one spot left to place and we know that the T column has exactly one checkmark, and this column has a checkmark already, so we know that T-F is an X.

These are the only two possibilities in the game. So with question 20, we need to see which hypothetical pertains to the condition, perhaps it is both.
To have the exact same S and T columns, we must go with the left one. And at that point, I would, in exponent-like fashion, place the respective checkmark at T-G inside of a little circle and an X at T-F inside of a little circle. After I am done with that question, I would erase those two circles. I do this only due to the fact that this game is so limited that erasing information is not an issue.
This question could be solved by looking at a global diagram. Mine is below:

For the S and T columns to be exactly the same, I must place an X for S-H and for T-F.
This would force me to place a checkmark for T-G, as I know that exactly one person votes for G, and there is only one person left. This would then force S-G to be a checkmark to keep with the condition of the question.
That is one way to solve it. Or you could, as I did from the beginning, frame the game. You know it has such limited options.
From the global diagram, as shown above, you can see that the R column must contain exactly 2 checkmarks, and we only have one person left to vote, which means R-H must have a checkmark.
Knowing that we have a constraint that each voter must have at least one checkmark and at least one X, we can see that only voter G has potential issues with this requirement, as everybody else has both checkmarks and X's. At this point, I would frame the game around S-G being a check mark and S-G being an X.

If you use the global constraints concerning the alottment of checkmarks and X's each column has, you can quickly infer that in the left hypothetical, S-H must be an X. In this same hypothetical, we only have two spaces left to figure out. We know one must be a checkmark and one must be an X. However, all of the other requirements have been met, which involved each voter having at least a checkmark and an X, so this means that we can have a dual option amongst these two variables.
In the right hypothetical, we can quickly infer two things. We know that the G row must have at least one checkmark, so this means that T-G will have a checkmark. We also know that the S column has exactly one checkmark, and we only have one voter left, so S-H is a checkmark. We have one spot left to place and we know that the T column has exactly one checkmark, and this column has a checkmark already, so we know that T-F is an X.

These are the only two possibilities in the game. So with question 20, we need to see which hypothetical pertains to the condition, perhaps it is both.
To have the exact same S and T columns, we must go with the left one. And at that point, I would, in exponent-like fashion, place the respective checkmark at T-G inside of a little circle and an X at T-F inside of a little circle. After I am done with that question, I would erase those two circles. I do this only due to the fact that this game is so limited that erasing information is not an issue.
2 posts Page 1 of 1